Differential calculus

Tangent to a curve

Curve with tangent line

Tangent function animation

The graph of y=-2x+13

Graph of the function f(x)=x^2 with a tangent line drawn to (2,4)

Differential calculus is a subfield of calculus that focuses on the study of how things change. It is one of the two traditional divisions of calculus, the other being integral calculus, which focuses on the accumulation of quantities. The primary object of study in differential calculus is the derivative. A derivative represents an instantaneous rate of change, offering a way to calculate the rate at which a quantity changes at a specific point.

Overview

Differential calculus deals with the calculation of derivatives and their applications. The derivative of a function at a chosen input value describes the rate of change of the function near that input value. The process of finding a derivative is called differentiation. The fundamental theorem of calculus links differentiation with integral calculus through the antiderivative.

History

The development of differential calculus, along with its counterpart integral calculus, is credited to Isaac Newton and Gottfried Wilhelm Leibniz in the 17th century. Their work on the calculus was built upon earlier contributions by mathematicians such as Pierre de Fermat, who developed methods for finding tangents to curves, a key concept in differentiation.

The Derivative

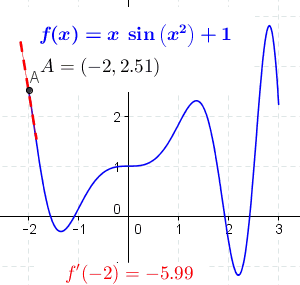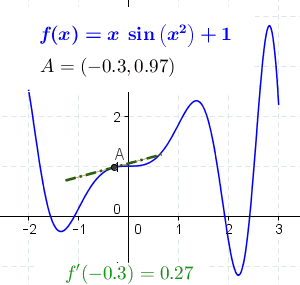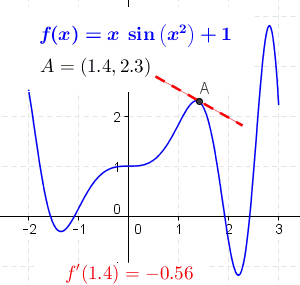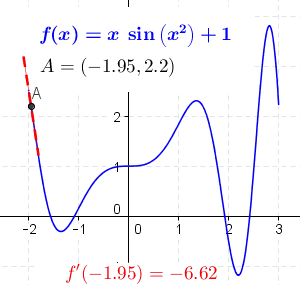
The derivative of a function measures how the function's output changes as its input changes. Formally, the derivative of a function f at a point x is defined as the limit of the ratio of the change in f(x) to the change in x as the change in x approaches zero. This is often written as:

\[ f'(x) = \lim_{\Delta x \to 0} \frac{f(x + \Delta x) - f(x)}{\Delta x} \]

If this limit exists, the function is said to be differentiable at the point x. The derivative itself is a function that gives the rate of change of the original function at each point in its domain.

Techniques of Differentiation

Several rules simplify the process of differentiation for various types of functions:

• The Power Rule: For any real number n, the derivative of x^n is nx^(n-1).
• The Product Rule: The derivative of the product of two functions is given by the product of the first function and the derivative of the second function plus the product of the second function and the derivative of the first.
• The Quotient Rule: The derivative of a quotient of two functions is given by the denominator times the derivative of the numerator minus the numerator times the derivative of the denominator, all divided by the square of the denominator.
• The Chain Rule: The derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function.

Applications

Differential calculus has wide-ranging applications in many fields including physics, engineering, economics, and biology. It is used to find the maximum and minimum values of functions, to study motion, and to solve optimization problems, among other things.

See Also

• Integral calculus
• Limit (mathematics)
• Function (mathematics)
• Tangent
• Optimization (mathematics)