Frequency domain

Fourier transform time and frequency domains (small)

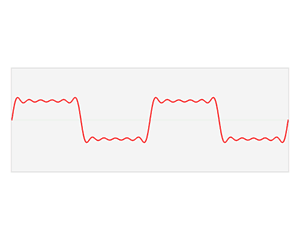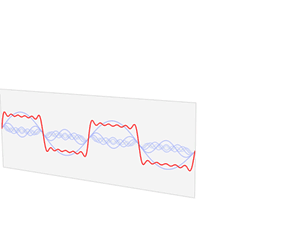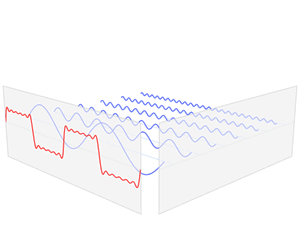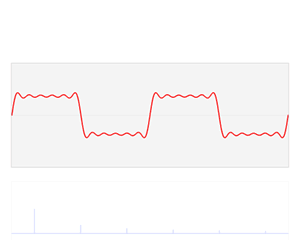
Frequency domain refers to the analysis of mathematical functions or signals with respect to frequency, rather than time. Essentially, it is a way of describing how the various components of a signal or system vary across different frequencies. This concept is fundamental in many areas of engineering, physics, and applied mathematics, particularly in fields such as signal processing, electromagnetic theory, and acoustics.

Overview

In the time domain, signals are represented as a function of time. However, analyzing these signals in the frequency domain provides insights into the signal's frequency components. This is achieved by transforming the time-domain signal into the frequency domain using mathematical transformations such as the Fourier transform. The result is a representation of the signal in terms of its constituent frequencies, often simplifying the analysis of complex signals.

Fourier Transform

The Fourier transform is a mathematical operation that transforms a time-domain signal into its frequency-domain representation. It decomposes a function of time (a signal) into the frequencies that make it up, similar to how a musical chord can be expressed as the frequencies of its constituent notes. The inverse Fourier transform can be used to convert the frequency-domain data back into the time domain, allowing for a seamless transition between the two representations.

Applications

The frequency domain analysis is widely used in various fields for different purposes:

- In signal processing, it helps in filtering, signal compression, and identifying the frequency components of a signal. - In acoustics, it is used to analyze the characteristics of audio signals and the acoustical properties of spaces. - In electromagnetic theory, it aids in understanding the behavior of electromagnetic waves across different frequencies. - In control systems, frequency domain methods are used for the design and analysis of systems to achieve desired performance characteristics.

Advantages

Analyzing signals in the frequency domain offers several advantages:

1. **Simplification of Problems**: Many problems that are complex in the time domain become simpler when analyzed in the frequency domain. 2. **Filter Design**: It is easier to design filters in the frequency domain to remove unwanted components from a signal. 3. **System Analysis**: The stability and response of a system can be more easily analyzed and understood.

Spectral Analysis

Spectral analysis is a key application of frequency domain techniques, involving the examination of the spectrum of frequencies or energy levels in a signal. This is crucial in identifying the dominant frequencies and their amplitudes, which can be critical in understanding the nature of the signal or system being analyzed.

Conclusion

The frequency domain offers a powerful perspective for analyzing signals and systems, complementing the time-domain analysis. By understanding the frequency components of a signal, engineers and scientists can design, analyze, and improve systems in various fields of technology and science.

This article is a stub. You can help WikiMD by registering to expand it. Editing is available only to registered and verified users. WikiMD is a comprehensive, free health & wellness encyclopedia.