Transpose

Matrix transpose

Row and column major order

Transpose refers to the operation of changing the order or position of elements within a mathematical matrix or a data structure. In the context of mathematics, to transpose a matrix means to flip a matrix over its diagonal, turning the matrix's row into columns and vice versa. This operation is fundamental in various areas of mathematics, linear algebra, computer science, and related fields, playing a crucial role in solving linear equations, transforming coordinate systems, and manipulating data in programming.

Definition

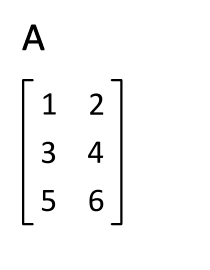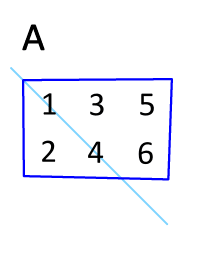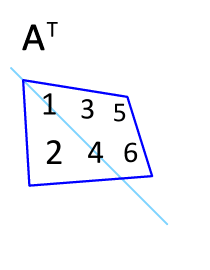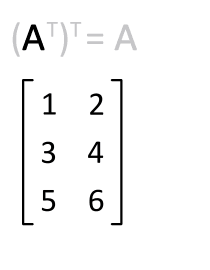
Given a matrix A of dimensions m × n, where m is the number of rows and n is the number of columns, the transpose of A, denoted as A^T or sometimes A', is a new matrix A^T of dimensions n × m where each element a_ij in A is mapped to a_ji in A^T. This means that the first row of A becomes the first column of A^T, the second row of A becomes the second column of A^T, and so on.

Applications

Transpose operations are widely used across different fields:

- In linear algebra, the transpose is used in the calculation of a matrix's determinant, in finding inverse matrices, and in the definition of symmetric matrices and orthogonal matrices. - In computer science, transposing data structures is a common operation in algorithm design and optimization, especially in the manipulation of arrays, data frames, and matrices for data analysis and machine learning. - In signal processing, transposing is used in the formulation and solution of systems of linear equations, which are fundamental in the analysis and interpretation of signals.

Properties

The transpose operation has several important properties: - **Symmetry**: If you transpose a matrix twice, you get back the original matrix. Mathematically, (A^T)^T = A. - **Inverse**: The transpose of the product of two matrices is equal to the product of their transposes in reverse order. That is, (AB)^T = B^TA^T. - **Addition**: The transpose of a sum of two matrices is equal to the sum of their transposes. That is, (A + B)^T = A^T + B^T.

Transpose in Programming

In programming, transposing a matrix or an array is a common task, which can be achieved through nested loops or, in higher-level languages, with built-in functions. For example, in Python, the NumPy library offers the transpose function, which can transpose arrays of any dimension.

See Also

- Matrix - Linear algebra - Computer science - Data structure