De Moivre–Laplace theorem

Quincunx (Galton Box) - Galton 1889 diagram

De moivre-laplace

De Moivre–Laplace theorem is a fundamental result in the field of probability theory and statistics that serves as a bridge between binomial distributions and the normal distribution. This theorem is named after Abraham de Moivre and Pierre-Simon Laplace, two prominent mathematicians who made significant contributions to probability theory and statistics.

Overview

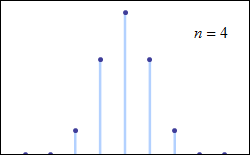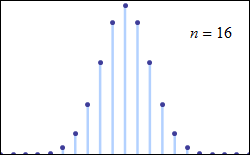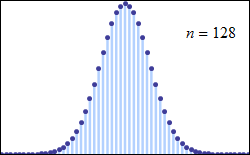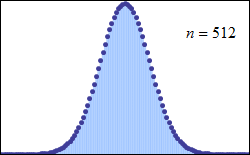
The De Moivre–Laplace theorem is primarily concerned with the approximation of binomial distributions. It states that as the number of trials in a binomial experiment increases, the shape of the binomial distribution approaches that of a normal distribution. Specifically, if an experiment consists of n independent trials, each with a probability p of success and q = 1 - p of failure, then the binomial probability distribution of the number of successes can be approximated by the normal distribution, provided that n is sufficiently large.

Mathematical Formulation

Mathematically, the theorem can be expressed as follows: If X is a binomial random variable with parameters n and p, then the standardized variable

\[ Z = \frac{X - np}{\sqrt{npq}} \]

converges in distribution to a standard normal variable as n → ∞. Here, np is the mean of the binomial distribution, and \sqrt{npq} is its standard deviation.

Applications

The De Moivre–Laplace theorem is widely used in various fields, including statistics, engineering, economics, and social sciences, to approximate probabilities of outcomes in binomial settings. It is particularly useful in situations where calculating binomial probabilities directly is computationally intensive or impractical due to a large number of trials.

Historical Context

Abraham de Moivre, a French mathematician, initially discovered the theorem in the 18th century. He published his findings in his work "The Doctrine of Chances," where he provided a method to approximate binomial probabilities using the normal curve. Later, Pierre-Simon Laplace expanded on de Moivre's work, further establishing the connection between binomial and normal distributions. Their combined efforts laid the groundwork for the central limit theorem, a cornerstone of probability theory.

See Also

• Binomial distribution
• Normal distribution
• Central limit theorem
• Probability theory
• Statistics

‎